Hi,
I’m hoping some of the cipher/math guys can tell me if this possible solution is statistically significant, or not. It’s Rand’s theory, and I’ve copied below from the Troy Houghton/Minutemen thread. I don’t want to discuss those potential suspects, I’m just hoping someone who really understands statistics can tell me if this potential solution is likely, or unlikely, random.
Thanks.
I’m very curious what people think of this. There is a lot of selective logic going on with this interpretation of the "unsolved 18" in that, first, many of the characters have several possible translations, and second, it’s an anagram.
Also, all of the letters which re-translate to mostly, or exclusively, non-letters are not included in the solution, but the letter "B" translates only to "V" which isn’t in the solution Rand came up with. Maybe it stands for "5", or maybe it’s more reason to say the theory doesn’t hold up. Or, maybe to Z, the "V" is supposed to be a symbol and not a letter, as on the Halloween card.
However, it does seem way beyond coincidence that such a message can be derived by this very logical method.
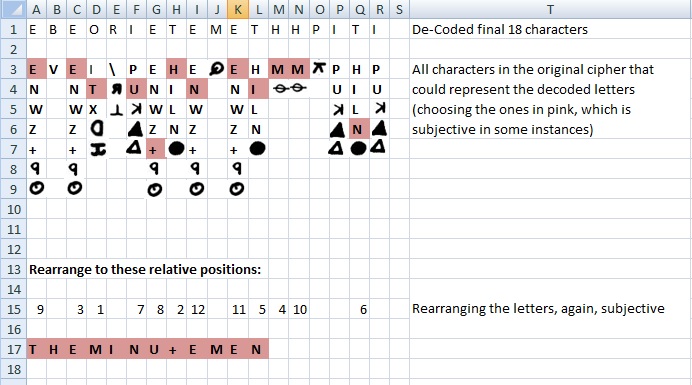
rand, Subject: The Minu+emen in the last line of the 408 Sun Apr 04, 2010 11:31 amZodiac’s first cipher was sent in three parts to three different Bay Area papers. Here are the three parts of the cipher:
part 1: http://www.zodiackiller.com/VTHCipher.html
part 2: http://www.zodiackiller.com/VTHCipher.html
part 3: http://www.zodiackiller.com/ExaminerCipher.htmlZodiac said his identity (not name) would be known once police cracked the cipher. It wasn’t. For forty years people have believed that Z lied about this. IMO, he didn’t lie.
Notice that the last line of the cipher is not part of the message (it isn’t translated).
When translated it reads: EBEORIETEMETHHPITI with the E coming from the last character of the second to last line.Gibberish, right? That’s what everyone has thought. But I believe that what Z did has not been understood. When it is, the last line, IMO, reads: THE MINU+EMEN with 5 extra symbols leftover that are not letters. When I say the last line reads, I mean the last line in the code part of the cipher, not the translation, which remains EBEORIETEMETHHPITI. Thus, Z’s identity is, as he said, in the code or cipher itself. But the code had to be cracked first, as he said, to see this. The trick is this:
What you do is take the translated letters — EBEORIETEMETHHPITI — and work backwards: that is, find the equivalents in the cipher for the letters. Of course, working backwards like this will give you exactly what the Zodiac orignally wrote on the last line. But it will also give you some other things as well.
Why? Because there are other characters that could have been used to yield the EBEORIETEMETHHPITI translation.
Why? Because some letters have two or more characters associated with them. When this is done, VOILA!, you get an anagram for +HE MINUTEMEN (a plus sign is used for one of the T’s) with six characters left over: BRMPII. (see below) All of the six characters are not letters, but rather shapes, e.g., V/+ ^. Thus, they cannot be used in the translation — a clever way for Z to say that only 12 of the 18 characters have meaning — the other five are filler to yield the rest of the 17-character line.
Thus,OTEHTEIEEHET BRMPII (TRANSLATED LINE)
THEMINU+EMEN +^Q/> (CODE LINE)The top line (which is the translation) is precisely the same as EBEORIETEMETHHPITI — just in a different order (because THE MINUTEMEN was anagrammed)
So Z’s identity was, IMO, revealed once the cipher was cracked.
I can’t give you numbers but I personally feel that it is overly ambigious. As doranchak has shown, just by anagramming alone, many viable combinations of words can be made. There is also strong statistical evidence that Zodiac pulled down fragments (4-gram, etc) from the cipher to fill in the last 18 characters so that all parts are even.
Starts around time index 6:45. https://www.youtube.com/watch?v=BV5R3TBMWJg
I don’t want to discuss those potential suspects, I’m just hoping someone who really understands statistics can tell me if this potential solution is likely, or unlikely, random.
Jarlve is correct. The one solution found by rand is simply one among an astronomically large number of possibilities.
Here are the symbols again:

Since rand permits the "+" to stand for "T", I am replacing all the "letter-like" symbols with their letter equivalents (for example, backwards R is counted as regular R):

Now, rand picks one letter from each column, so let’s count how many choices there are in each column:
Col 1: 7 choices
Col 2: 1 choice
Col 3: 7 choices
Col 4: 5 choices
Col 5: 3 choices
Col 6: 3 choices
Col 7: 7 choices
Col 8: 5 choices
Col 9: 7 choices
Col 10: 1 choices
Col 11: 7 choices
Col 12: 5 choices
Col 13: 2 choices
Col 14: 2 choices
Col 15: 1 choices
Col 16: 3 choices
Col 17: 5 choices
Col 18: 3 choices
Let’s consider an 18-letter sequence chosen from those columns. How many different ways can you make these choices? Answer: There are 7 * 1 * 7 * 5 * 3 * 3 * 7 * 5 * 7 * 1 * 7 * 5 * 2 * 2 * 1 * 3 * 5 * 3 = 3,400,000,000 (3.4 billion) choices.
So, before you even get to the anagramming step, you have 3.4 billion options to pick from!
Now, let’s say you pick one of those 3.4 billion 18-letter sequences. How many ways can you rearrange the 18 letters? Answer: 18*17*16*…*2*1 = 18 factorial = 6,402,373,705,728,000 (6.4 quadrillion, or 6.4 million billion)
Since there are 3.4 billion sequences, and 6.4 quadrillion anagrams per sequence, the total number of anagrams to pick from is:
3.4 billion TIMES 6.4 quadrillion = 22,000,000,000,000,000,000,000,000 = 22 septillion (or 21 million billion billion). A portion of those will be duplicates due to repeated letters. But the number of possibilities is still astronomically large.
What if you could only find a good anagram at a rate of once per one trillion of those possibilities? Then you’d still have 22,000,000,000,000 (22 trillion) good anagrams to pick from!
So you can see, it’d be pretty easy to find lots of interesting words and phrases among the possibilities.
Thanks for your replies. I’m ready to dismiss the theory.
I do want to point out though, that your analysis about astronomical solutions is, I think, over-inflated. First, there are only 13 different letters, only 3 vowels, and several of the available letters are not very common (V, W, X, Z for example.) Many common letters are not available (A, C, D, O, R, S being the main ones.) So possible solutions would be severely limited, given all the words requiring one of those missing common letters, and the relatively few words using the uncommon ones. Any sequence with two or three of those 4 very uncommon letters next to each other would probably not result in any semblance of a meaningful solution.
Also, Rand’s solution is not a meaningless phrase, but the identity of a possible suspect (and an identity that would not give Z away.) It’s not something short, like Name Kane, or Gyke, but the 12-letter name of an organization. I’m not suggesting Z was The Minutemen, or even that Z was using misdirection to point at them… My question was purely about such a long solution, not totally but reasonably precise, using a limited selection of letters, just 3 vowels, and so on.
Anyway, again I appreciate your thoughtful responses. You’re probably right about it being flawed/ambiguous.
Yes, those are very fair points. Removing the effects of the repeated letters takes some extra effort. Here is an example:
Rand’s solution comes from an anagram of these 12 letters: EETUTHNEIMMN. So, there are (12 factorial = 479,001,600) rearrangements. But the repeated letters (3 E’s, 2 M’s, 2 N’s and 2 T’s) result in duplicated rearrangements. So the total count is adjusted as follows: 12! / (3! * 2! * 2! * 2!) = 9,979,200.
Thus instead of almost 500 million rearrangements, there are actually only about 10 million unique rearrangements of those 12 letters.
Still a lot. The count varies depending on which letters you start with, but you still have to account for all the possible ways to select some number of letters from the "pile." Then, in rand’s example, you’d have to count the number of ways to select 12 columns from the 18 (If I’m not mistaken, it’d be c(18,12) = 18!/(12!*(18-12)!) = 18,564. And finally, you’d have to count all the ways to select symbols from each of those 18,564 column-combinations.
I’m very confident that a high number of other "significant-seeming" context-specific phrases can be generated out of all these possibilities. It’d be an interesting but tedious exercise to actually produce them.

